I. Introduction & Overview
II. Results & Analysis
III. Conclusion
I. Introduction & Overview
Many traders and even professional institutions may make decisions based on the day of the week. Some may refuse to buy into new trades on Fridays or Mondays, claiming that the market tends to behave certain ways on certain days. Is this a valuable practice? The purpose of this test is to determine any statistical significance in the percent change and volatility of the market depending on the day of the week.
This backtest will begin on 01/01/2003 and will be performed on the SPDR S&P 500 ETF Trust (SPY). Percent change will be calculated using closing prices and the measurement of volatility will be each day's True Range measured as a percentage of price. True Range describes the nominal dollar amount that a security has moved in a day, and since price slowly increases over time, the True Range value is divided by the closing price to provide a "True Range Percentage of Price" metric. After summary statistics for each day of the week is reported, one-sided ANOVA tests will be performed to evaluate any statistical significance in the mean percent change in price and the mean true range percentage. The null hypothesis will state that all means are equal, while the alternative hypothesis will state that at least one mean is not equal to the others. The significance level will be a standard α = 0.05.
II. Results & Analysis
When running summary statistics for each day of the week on the SPDR S&P 500 ETF Trust (SPY) since 01/01/2003, the results are the following:
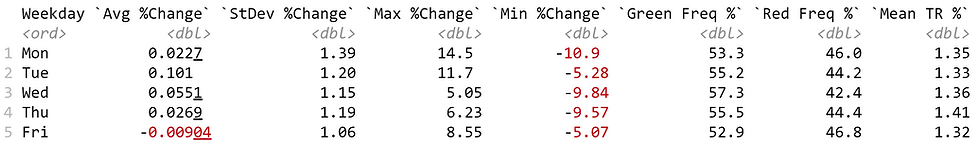
While empirical observations and conclusions can be made, it is important to test for statistical significance to determine the probability that these results are products of chance and randomness. When running ANOVA tests, comparing means in percent change produced a p-value of p = 0.3495 and comparing means in true range percentage produced a p-value of p = 0.4673. Therefore, we fail to reject the null hypothesis to conclude that there is any statistically significant difference in percent change or true range percentage across each day of the week. When analyzing each pair-wise comparison using Tukey's method, the results are the following:
This rows above show each pair-wise comparison, their difference in means, their lower and upper bounds for confidence intervals (95% CI) and their reported p-values. It is important to note that all p-values are far from α < 0.05 significance level. An interesting observation is that Fri-Tue comparison with the lowest p-value of 0.27, but still remains distant from any dependable conclusion. The market's heavy random walk makes finding an edge more difficult than something as simple as trading based on the day of the week and keeps mean returns close to zero.
III. Conclusion
While a simple statistical summary for each day of the week may produce results that seem significant by observation, the ANOVA results suggest that there is a large probability that the differences are due to randomness. It is unlikely that making decisions solely based on the day of the week will add or diminish any significant value to returns.





Comments